One Sample t-Test (Jump to: Lecture | Video )

Let's perform a one sample t-test: In the population, the average IQ is 100. A team of scientists wants to test a new medication to see if it has either a positive or negative effect on intelligence, or no effect at all. A sample of 30 participants who have taken the medication has a mean of 140 with a standard deviation of 20. Did the medication affect intelligence? Use alpha = 0.05.

Steps for One-Sample t-Test
1. Define Null and Alternative Hypotheses 2. State Alpha 3. Calculate Degrees of Freedom 4. State Decision Rule 5. Calculate Test Statistic 6. State Results 7. State Conclusion

Let's begin.

1. Define Null and Alternative Hypotheses

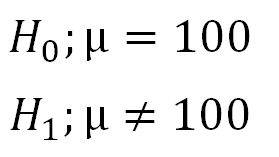
Figure 1.

2. State Alpha

Alpha = 0.05

3. Calculate Degrees of Freedom

df = n - 1 = 30 - 1 = 29

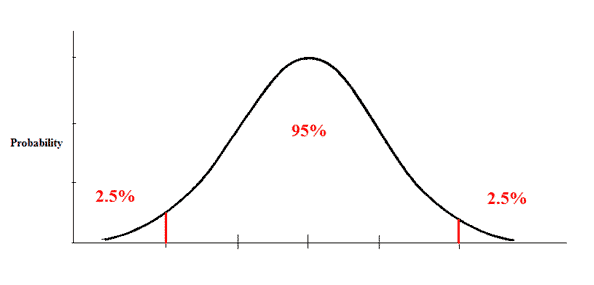
4. State Decision Rule

Using an alpha of 0.05 with a two-tailed test with 29 degrees of freedom, we would expect our distribution to look something like this:

Figure 2.

Use the t-table to look up a two-tailed test with 29 degrees of freedom and an alpha of 0.05. We find a critical value of 2.0452. Thus, our decision rule for this two-tailed test is:

If t is less than -2.0452, or greater than 2.0452, reject the null hypothesis.

5. Calculate Test Statistic

Figure 3.

6. State Results

t = 10.96

Result: Reject the null hypothesis.

7. State Conclusion

Medication significantly affected intelligence, t = 10.96, p < 0.05.

---